Calculations§ were performed at the B3LYP Density functional ab initio level,4 using a Double Zeta all-electron basis set with polarisation functions (DZVP) for all the elements which has been show to result in realistic geometries and energies for complexes of palladium.5 The aromaticity at the ring centroid was estimated via the GIAO-NICS method6.
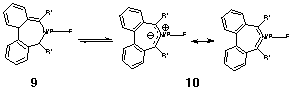
Considering first the computed properties of 1 and 2 (Table 1), we note that the Huckel-aromatic ring in 2 exhibits a typically aromatic NICS value which is perturbed only slightly from the value for the unbound ligand 1. The ring bond lengths in 2 show little alternation, properties which are essentially unperturbed by coordination to Pd. The displacement of one PMe3 ligand from the metal by 1 (Scheme) is moderately exothermic by 9.4 kcal/mol

The homologue 3 in comparison has a NICS value indicative of significant anti-aromaticity, reduced by 4.2 ppm (i.e. less anti-aromatic) when the formal carbene is coordinated to the Pd. This is accompanied by a 40 increase in the degree of C2 twisting of the ligand associated with the greater degree pf Möbius character.3 The energy of PMe3 displacement (Scheme) is largely unperturbed compared to 1, indicating that 3 should be as effective as 1 in displacing phosphine ligands. Subsequent modelling was with PH3 replacing PMe3. This approximation results in over-estimating the phosphine displacement energy by about 4 kcal/mol (Table 1), since the decreased electron donating capability of PH3 makes it a less effective ligand.
With R',R"=H as the ring substituent, the barrier to inversion of the ring in 4 is very small, as we had previously noted.2 Our next objective therefore was to investigate if this barrier could be increased to the point where thermal racemisation of an enantiomerically pure ligand could be inhibited. The first system we investigated, R',R"=F is known to have the effect of significantly increasing the Möbius-aromaticity of the ligand.2 The NICS value of the free ligand (0.3 ppm) makes it in effect non-aromatic, and the degree of twist as measured by one ring dihedral increases to 35o. When metal-bound, only a small change in the NICS to a slightly negative is computed. The inversion barrier both for the free and metal-bound ligand is now a significant 7 kcal/mol. Commensurate with the increased Mobius aromaticity (reduced Huckel anti-aromaticity) due to the ligand twist, the ring bond lengths show a small reduction in their alternation in both cases.
Introducing steric constraints (R'=Me) has as expected a larger effect on the inversion barrier and the degree of twisting (43o) but less effect than R=F on reducing the anti-aromaticity, and as before, no significant effect on the energy of ligand displacement. A synthetically more realistic ligand (5) where the steric constraint is provided by two benzo groups, reveals a thermally high barrier to inversion (and one that could be further increased by appropriate substitution on the benzo group) for the metal bound complex 6. The final variation we investigated was replacing the carbene centre by a silylene (Z=Si). Examples of such substitution are known for 1 with metals such as Ni and Pt,7 although surprisingly apparently not for Pd. The 8 p electron silyl homologue appears to exhibit a further small increase in the Möbius-aromaticity of the ring, whilst again having a very similar ligand displacement energy and ring inversion barrier to the carbon analogue.
We conclude that these carbene and silylene ligands are of an unusual class where their geometry (and hence chirality) is induced by an aromatising trend as a consequence of their partial 8 p electron Möbius aromaticity3 and which we suggest could form the basis for engineering potentially chiral monodentate metal coordination.
§Electronic Supplemental information (ESI) for this article is available at http://www.rsc.org/...
References
- See for example W. A. Herrmann and C. Kocher, Angew. Chem. Int. Ed. Engl., 1997, 36, 2162-2187; D. Bourissou, O. Guerret, F. P. Gabbï and G.Bertrand, Chem. Rev., 2000, 100, 39-91; W. A. Herrmann, V. P. W. Bohm, C. W. K. Gstottmayr, M. Grosche, C. P. Reisinger and T. Weskamp, J. Organomet. Chem., 2001, 617-618, 616-628.
- C. Kastrup, S. V. Oldfield and H. S. Rzepa, ChemComm., 2002, 642; W. L. Karney, C. J. Kastrup, S. P. Oldfield and H. S. Rzepa, J. Chem. Soc., Perkin Trans 2, 2002, 388-392. S. Martin-Santamarêa and H S. Rzepa, J. Chem. Soc., Perkin Transactions 2, 2000, 2372-2377; S. Martên-Santamarêa, and Henry S. Rzepa, ChemComm., 2000, 1089.
- E. Heilbronner, Tetrahedron Lett., 1964, 4, 1923.
- Gaussian 98 (Revision A.8), M. J. Frisch, G. W. Trucks, H. B. Schlegel, G. E. Scuseria, M. A. Robb, J. R. Cheeseman, V. G. Zakrzewski, J. A. Montgomery, Jr., R. E. Stratmann, J. C. Burant, S. Dapprich, J. M. Millam, A. D. Daniels, K. N. Kudin, M. C. Strain, O. Farkas, J. Tomasi, V. Barone, M. Cossi, R. Cammi, B. Mennucci, C. Pomelli, C. Adamo, S. Clifford, J. Ochterski, G. A. Petersson, P. Y. Ayala, Q. Cui, K. Morokuma, D. K. Malick, A. D. Rabuck, K. Raghavachari, J. B. Foresman, J. Cioslowski, J. V. Ortiz, A. G. Baboul, B. B. Stefanov, G. Liu, A. Liashenko, P. Piskorz, I. Komaromi, R. Gomperts, R. L. Martin, D. J. Fox, T. Keith, M. A. Al-Laham, C. Y. Peng, A. Nanayakkara, C. Gonzalez, M. Challacombe, P. M. W. Gill, B. G. Johnson, W. Chen, M. W. Wong, J. L. Andres, M. Head-Gordon, E. S. Replogle and J. A. Pople, Gaussian, Inc., Pittsburgh PA, 1998. DZVP basis sets; N. Godbout, D. R. Salahub, J. Andzelm and E. Wimmer, Can. J. Chem., 1992, 70, 560. Basis set for palladium: N. Godbout, PhD Thesis, 1992, Dept. Chim., University of Montreal, Quebec, Canada. Basis sets were obtained from the Extensible Computational Chemistry Environment Basis Set Database, Version 11/29/01, as developed and distributed by the Molecular Science Computing Facility, Environmental and Molecular Sciences Laboratory, Pacific Northwest Laboratory, P.O. Box 999, Richland, Washington 99352, USA. See http://www.emsl.pnl.gov:2080/forms/basisform.html
- M. Jakt, L. Johannissen, H. S. Rzepa, D. A. Widdowson and R. Wilhelm, Perkin Trans. 2, 2001, 576-581.
- For references to the NICS technique, see P. v. R. Schleyer, C. Maerker, A. Dransfeld, H. Jiao, and N. J. R. van Eikema Hommes, J. Am. Chem. Soc., 1996, 118, 6317; H. Jiao and P. v. R. Schleyer, J. Phys. Org. Chem., 1998, 11, 655.
- Ni: T. A. Schmedake, M. Haaf, B. J. Paradise, D. Powell, R. West, Robert. Organometallics, 2000, 19, 3263-3265; Pt: B. Gehrhus, P. B. Hitchcock, M. F. Lappert, H. Maciejewski, ibid, 1998, 17, 5599-5601.
| Table 1. Computed B3LYP/DZVP Energies, Geometries and NICS values for 1-4.§ | |||||
|---|---|---|---|---|---|
| Structure; Substituents | Energy, Hartree (B3LYP/dzvp) | NICS, ppm | Geometrya | DE Coordination (Kcal/mol)b | |
| 1; R=CH3 | -304.8242 | -11.8 | 1.37,1.39,1.36; 0.0 | - | |
| 2; R=CH3, L=P(Me)3 | -5705.7038 | -10.8 | 1.37,1.39,1.36,2.13; 0.0 | -9.4 | |
| 3; Z=C,R,R',R"=H | -303.5733 | 19.2 | 1.36,1.43,1.34,1.47; 18.45 | - | |
| 4; Z=C, R,R',R"=H, L=P(Me)3 | -5704.4513 | 14.7 | 1.37,1.43,1.34,1.47,2.10; 22.43 | -8.4 | |
| 4; Z=C, R,R',R"=H, L=PH3 | -5586.4758 | 15.0 | 1.36,1.43,1.34,1.47,2.12; 22.69 | -12.5 | |
| 4; Z=C, R,R',R"=H, L=PH3 | 0.5c | 22.7 | 1.36,1.43,1.34,1.47,2.12; 0.0 | - | |
| 3; Z=C, R=H, R',R"=F | -700.5951 | 0.3 | 1.37,1.41,1.34,1.45; 34.68 | - | |
| 4; Z=C, R=H,R',R"=F, L=PH3 | -5983.4949 | -1.4 | 1.37,1.41,1.34,1.45,2.08; 34.06 | -10.8 | |
| 4; Z=C, R=H, R',R"=F, L=PH3 | 7.1c | 12.2 | 1.36,1.42,1.34,1.47,2.10; 0.0 | - | |
| 3; Z=C, R,R',R"=CH3 | -539.4585 | 5.6 | 1.37,1.45,1.35,1.48; 47.59 | - | |
| 4; Z=C, R,R',R"=CH3, L=PH3 | -5822.3607d | 3.9 | 1.37,1.45,1.35,1.48,2.15; 46.16 | -12.3 | |
| 5; R=CH3 | -689.5161 | 6.9 | 1.37,1.44,1.41,1.48; 45.36 | - | |
| 6; R=CH3, L=PH3 | -5972.4158 | 5.3 | 1.37,1.44,1.41,1.48,2.14; 43.31 | -10.7 | |
| 6; R=CH3, L=PH3 | 21.7c | 11.5 | 1.36,1.45,1.43,1.51,2.28; 0.0 | - | |
| 3; Z=Si,R,R',R"=H | -555.0256 | 12.5 | 1.75,1.42,1.35,1.47; 25.15 | - | |
| 4; Z=Si, R,R',R"=H, L=PH3 | -5837.9274 | 9.9 | 1.74,1.42,1.35,1.47,2.30,; 25.70 | -12.0 | |
| 4; Z=Si,R,R',R"=H, L=PH3 | 0.5c | 14.8 | 1.73,1.42,1.35,1.47,2.30; 0.0 | - | |
| 3; Z=Si, R=H,R',R"=F | -952.0553 | -0.8 | 1.77,1.39,1.34,1.45; 41.40 | - | |
| 4; Z=Si, R=H, R',R"=F, L=PH3 | -6234.9566 | -2.2 | 1.75,1.39,1.34,1.45,2.27; 40.79 | -11.7 | |
| 4; Z=Si, R=H,R',R"=F, L=PH3 | 7.9c | 8.1 | 1.74,1.40,1.34,1.40,2.27; 0.0 | - | |
a Geometric data: bond length, Å (C2-N3, N3-C4, C4-C5, C5-C6, and Pd-C2 for metal ligand complex); dihedral angle (C4-C5-C6-C7). bFor the equilibrium shown in scheme 1. c Energy of planar form relative to C2 geometry, in kcal mol-1. d Because of the geared methyl interactions, a formal transition state for inversion of chirality proved difficult to locate. We estimate the barrier to be > 30 kcal/mol on the basis of constraining the ring atoms to co-planarity.