In the previous post, the molecule F3S-C≡SF3 was found to exhibit a valence bond isomerism, one of the S-C bonds being single, the other triple, and with a large barrier (~31 kcal/mol, ν 284i cm-1) to interconversion of the two valence-bond forms. So an interesting extension of this phenomenon is shown below:

A cyclic form of the SCS Motif. Click for 3D
A B3LYP/cc-pVTZ calculation (DOI: 10042/to-3646) reveals that the optimized geometry exhibits six equal SC bonds, all 1.616Å long. Typically, a single SC bond is around 1.82Å, a double 1.65Å and a triple is about 1.5Å at the same level of theory, so this C=S bond is clearly at least a double one. A NICS(0) calculation at the centroid has the value of -14.6 ppm, which indicates aromaticity. We conclude the appropriate arrow above is the bottom resonance one, rather than the top equilibrium one. This is confirmed by finding that the Kekulé vibrational mode has a strongly positive force constant (ν 1083 cm-1, animated in 3D model above), which contrasts with the negative value (ν 284i cm-1) found for bond shifting in F3S-C≡SF3 itself. Again, comparison indicates that a C≡S triple bond has a frequency of around 1400 cm-1 and a double around 1200 cm-1 (the degenerate C=S non–Kekulé vibrational mode for this system is indeed calculated at around 1225 cm-1). So to summarise; a single F3S-C≡SF3 unit reveals very strong bond alternation, and negative force constant (transition state) for interconversion of the two bond forms, but a cyclic form reveals the opposite behaviour, with no alternation and instead strong aromaticity.
In part this difference in behaviour must be due to the constraints on the geometry of the cyclic form. F3S-C≡SF3 interconverts via a highly twisted geometry with C2 symmetry, and this twisting is not exactly possible if you create a cyclic equivalent. In part it is also due to the aromatic stabilisation energies. In the resonance above, you should be able to count a total of 12 electrons involved! Nominally, if you try to apply the 4n+2 aromaticity rule, it does not fit, until you realise that in fact you must be dealing with two sets of 6 electrons. The system in fact is a classic double-aromatic, in which six electrons circulate in the plane of the molecule (the σ-set) and six above and below (the π-set; the MOs for the molecule confirm exactly this interpretation). Notice how this itself contrasts with a similarly aromatic system, the atom swapping in three nitrosonium cations, where the Kekulé mode force constant was strongly negative.

ELF Analysis for F6S3C3. Click for 3D
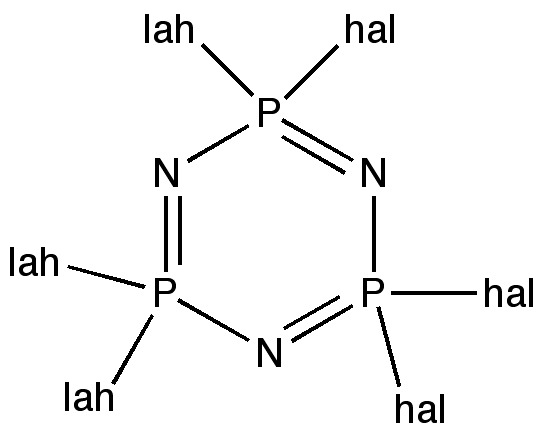
All that is needed is is for someone to make this molecule to confirm its properties. Perhaps by trimerising F2SC, itself formed by cheletropic elimination? It is worth noting that the iso-electronic P/N (e.g. of S/C) analogues are very well known.
Tags: aromaticity, Hypervalency, Interesting chemistry, pericyclic, South Carolina

Is the sulfoxide version (in SMILES, C1#S(=O)C#S(=O)C#S(=O)1) also predicted aromatic? That seems as though it would be easier to synthesize.